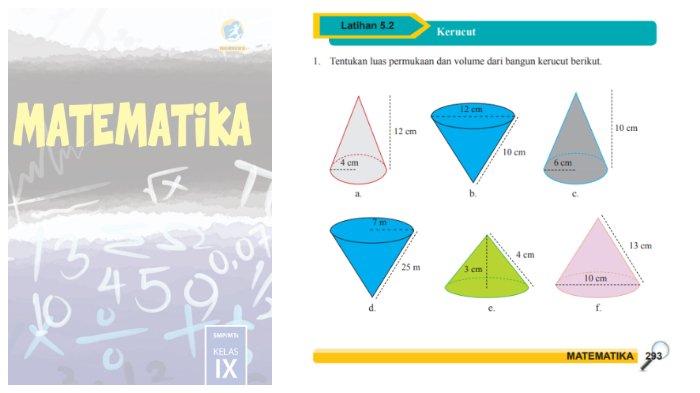
Kunci Jawaban Matematika Kelas 9 Halaman 293 294 295 296 K13: Luas Permukaan dan Volume Kerucut
Kunci jawaban Matematika Kelas 9 halaman 293 294 295 296 K13 hitung luas permukaan dan volume kerucut, Latihan 5.2, Semester 2, dapat menjadi koreksi.
Penulis: Muhammad Alvian Fakka
Editor: Facundo Chrysnha Pradipha
Volume Kerucut = ⅓ πr²t
Volume Kerucut = ⅓ x 3,14 x 6² x 10
Volume Kerucut = 376,8 cm³
d.
t = √(s² - r²)
t = √(25² - 7²)
t = √(625 - 49)
t = √576 = 24
Luas Permukaan Kerucut = πr(r + s)
Luas Permukaan Kerucut = 22/7 x 7(7 + 25)
Luas Permukaan Kerucut = 22(32)
Luas Permukaan Kerucut = 704 cm²
Volume Kerucut = ⅓ πr²t
Volume Kerucut = ⅓ x 22/7 x 7² x 24
Volume Kerucut = 1232 cm³
e.
r = √(s² - t²) r = √(4² - 3²)
r = √(16 - 9) r = √7
Luas Permukaan Kerucut = πr(r + s)
Luas Permukaan Kerucut = 3,14 x √7(√7 + 4)
Luas Permukaan Kerucut = 8,31(6,65)
Luas Permukaan Kerucut = 55,23 cm²
Volume Kerucut = ⅓ πr²t
Volume Kerucut = ⅓ x 22/7 x (√7)² x 3
Volume Kerucut = 22 cm³
f.
t = √(s² - r²)
t = √(13² - 5²)
t = √(169 - 25)
t = √144 = 12
Luas Permukaan Kerucut = πr(r + s)
Luas Permukaan Kerucut = 3,14 x 5(5 + 13)
Luas Permukaan Kerucut = 15,7(18)
Luas Permukaan Kerucut = 282,6 cm²
Volume Kerucut = ⅓ πr²t
Volume Kerucut = ⅓ x 3,14 x 5² x 12
Volume Kerucut = 314 cm³
2. Tentukan panjang dari unsur kerucut yang ditanyakan.
Kunci Jawaban:
a.
Volume Kerucut = ⅓ πr²t
t = V x 3 : (πr²)
t = 300π x 3 : (π x10²)
t = 900 : 100 = 9 m
b.
Volume Kerucut = ⅓ πr²t
r² = (V x 3) : πt
r² = (120π x 3) : π10
r² = 360π : 10π
r² = 36
r = √36
r = 6 m
c.
Luas Permukaan Kerucut = πr(r + s)
(r + s) = Luas Permukaan Kerucut : (πr)
(8 + s) = 180π : (π8)
(8 + s) = 22,5
s = 22,5 - 8
s = 14,5
